- Home
- Photoshop ecosystem
- Discussions
- Re: Need to enlarge photo for 24' backdrop - help!
- Re: Need to enlarge photo for 24' backdrop - help!
Need to enlarge photo for 24' backdrop - help!
Copy link to clipboard
Copied
Hi,
I'm looking to enlarge a photo for a 24' backdrop. The stock photos are just not large enough to provide the printer with a file that's 1/2 size (146"w) at 72 dpi. When enlarging the photos in PS to the dimensions the biggest one I found was only 42 dpi. Check out the screen shot.
I remember someone at my previous job using a program to rez up images. Anyone know what that is? Any other ideas?
Thanks all!
Dydee
Explore related tutorials & articles

Copy link to clipboard
Copied
Copy link to clipboard
Copied
Copy link to clipboard
Copied
42PPI my be acceptable for a large image that's viewed from a distance - check with your printer.
Copy link to clipboard
Copied
Hi dydes57085107, When I open this posted photograph, I read the size at
64.2
159.214 inches wide
77 inches high
res only 42.779
If you are able to resave this at a higher resolution how far can you enlarge it?
Free transform might let you do it inside the current work-frame. Someone here might know the exact formula you need. Best always, JH
Copy link to clipboard
Copied
Copy link to clipboard
Copied
Hi
What distance will you view the backdrop from? You may not need higher than 42ppi. The resolution required can be calculated from 6878/Viewing distance in inches *.
So if your are viewing this from say 15 feet away (which is 180 inches) the ppi required is 6878/180 = 38 ppi. Any higher and your eyes cannot resolve it at that distance.
Dave
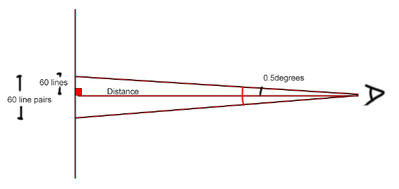
The math is based on this:
A good human eye can resolve 60 line pairs per degree i.e. pairs of black and white lines. So in half a degree we would have 60 single lines.
Simple trigonometry means that the width containing those 60 lines can be calculated by:
Width of 60 lines = Viewing distance x tan(0.5°)
Once we know the width for 60 lines (or pixels) we can calculate how many lines per inch can be seen by using 60/Width of 60 lines.
So putting those together gives us
60/(Viewing distance x tan(0.5°)) = 60/(Viewing distance x 0.0087269)
= 2/(Viewing distance x 0.000291)
Or
1/(Viewing distance x .0001454)
Or
6878/Viewing distance
Copy link to clipboard
Copied